AUTOMOTIVE CFD FOR DETERMINING AERODYNAMIC DESIGN COEFFICIENTS

A rather unorthodox concept car geometry was chosen for automotive CFD analysis and the force Coefficients were calculated. 

Figure No.1: STL geometry

Turbulence properties were calculated from the wheel base length of the car and the Aerodynamic coefficients were calculated from the frontal area of the car. Here are the steps adhered to calculate the frontal area of the car using paraview.

Here is a brief video tutorial on how to calculate the projection Area or the frontal area of a car.

 

The projection area and the wheelbase length values are called inside the force-coefficient file, within the system folder.

 Figure No.2: lRef(base length) & Aref(Projection Area)

The simulation was performed on a grid whose dimensionless wall-distance value was less than 1.0. A kOmegaSST turbulence model was adopted for a steady-state solver. 

Figure No.3: Meshed STL geometry

Along with the car, the ground patch or the lowerWall is also considered as a 'wall' patch and there are 3 region refinements adopted. There is refinement immediately around the car, a refinement for the ground and a refinement for the wake region. 

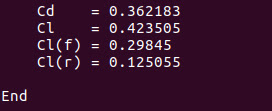
The simulation was run for 1500 iterations before the solver converged to a steady value of force-coefficients. 
 Figure No.4: Force-Coefficients


The residuals of the flow variables also satisfied the convergence criteria.
 Figure No.5: Residual Plot

The pressure contour below indicates the stagnation pressure and the overall pressure distribution.

 Figure No.6: Residual Contour

The flow streamline around the car geometry was also captured and illustrated below.

Figure No.7: Streamlines 

 The other plots are illustrated below.

 Figure No.8: The k-plot & nut-plot 

A good mesh is crucial to predict the right force-coefficient values.




Comments

Post a Comment

Popular posts from this blog

snappyHexMesh tutorial for a simple Geometry

Image
In this section I will address how to use the snappyHexMesh tool to mesh a Cylinder. We can either create an stl file for the cylinder - place it inside the  triSurface/constant  folder and call the same into the background mesh, or a simple cylinder can be directly created by using the 'searchable cylinder' parameter within the snappyHexMeshDict.  cylinder     { type searchableCylinder;   point1 (0 0.0 -0.5);  point2    (0 0.0 0.5); radius 0.01585;     } A refined mesh can be viewed below. The radius of the cylinder is of the order e-02 which gives us some insight into what the cell size for the back ground mesh can be. The blockMesh for the background mesh will be addressed later. Next in line in the snappyHexMeshDict is the Refinement box parameter. This box defines how refinement around the cylinder and importantly how the wake refinement can be achieved. The refinement box separates the finer ref...
Read more

snappyHexMesh Tutorial For a Complex Geometry and External Aerodynamics

Image
The geometry chosen for external fluid dynamics simulation is that of an offshore structure. In that, a deep-draft semi-submersible structure  is scaled scaled down to a value  of 0.01 (scaled down by a factor of 100). The semi-submersible structures are employed at locations where the oil extraction from the sea bed is considerably deep. These are movable structures which are half submerged during application. A working deck comes right on top of the structure where the cranes and oil rigs are fitted. The semi-submersible consists of four hull appendages which connect the working deck with a pontoon structure. The pontoon structure gives buoyancy to the semi-submersible in deep waters but does not come directly in contact with the ocean bed.  Figure No.1: DDS The effects of flow induced vibration on the above four columns during high flow currents is indeed the case of interest. Once a good mesh is achieved, the platform is set for the study on the effects...
Read more

AIRCRAFT OpenFOAM SIMULATION (BOEING 747)

Image
Before I begin to furnish the results, a quick look at the computational resources that were utilized for the simulation. I possess a minimal capability laptop manufactured by Hewlett-Packard, which runs on 2 core(s) and a 4 GB RAM. The objective of the simulation is to set up a 3D mesh for an Aircraft flow simulation with a resolved boundary layer of Y+ < 1, and to study the Residuals . The kOmegaSST turbulence model was adopted. The STL file downloaded online is a scaled Boeing 747 model, with the elevator tabs deflected downwards. Figure No.1: Rear View The cell size in the blockMesh is 0.05, owing to the fact that a scaled 747 model was selected, otherwise a cell size of 0.5 can be executed for an un-scaled model. Regardless, any of the following cell size can be applied (0.1, 0.2, 0.3, 0.4, 0.5, 0.6, 0.7, 0.8, 0.9, 1.0). Four boundary layers were generated with an expansion ratio of 1.2,  adding to the total cell count of over 3 million cells. A steady-state so...
Read more